Waveguide Array Transports Light Without Distortion
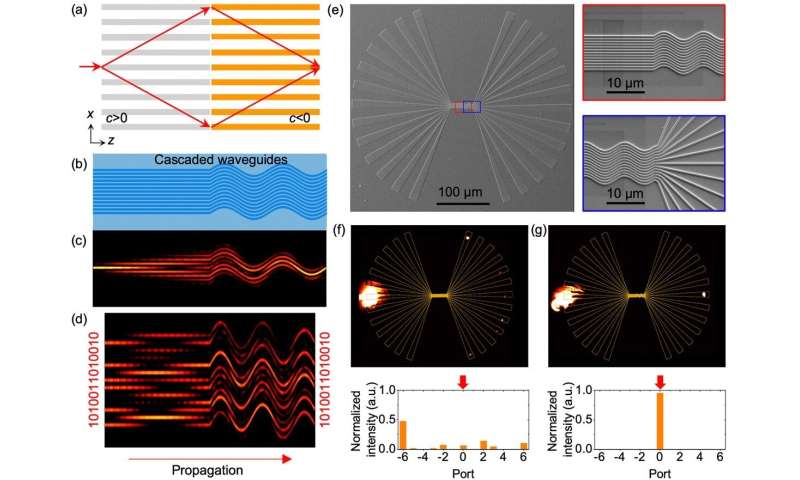
Subwavelength self-imaging in cascaded waveguide arrays. (a) Compensated positive and negative coupling in waveguide array for superlens. (b) Cascaded waveguides, and corresponding result of the simulated field evolution in (c). (d) Simulated signal results of “0”/“1” coded signal transmission through cascaded waveguide arrays. The output in cascaded waveguides perfectly reproduces the input signal. (e) SEM figures of the fabricated cascaded samples. CCD recorded optical propagation from input to output through (f) straight and (g) cascaded waveguide arrays. Credit: SPIE
One of the challenges of optical microscopy is to continually increase the imaging power, or resolution. In the past three hundred odd years, scientists have been building ever-better microscopes. The limit, for a long time, was determined by only two factors: the contrast of the object being viewed, and the resolving power of the optics in the microscope. The last 50 years, in particular, have led to an explosion in techniques to improve both the contrast of object and the quality of the optics.
One such technology is called a superlens. The superlens makes use of some of the peculiarities of waves to be able to resolve details that would otherwise be hidden from view. Now, researchers from Nanjing University in China have published results on a waveguide array that provides many of the benefits of a superlens. Along with that, the waveguide array does not have the technological difficulties that are usually associated with superlens fabrication.
That lens is super
To understand the superlens, it helps to understand how an image is formed. Let's begin with something like the head of a pin against a featureless background. When light is shone on the pin, it scatters in all directions. The details of the image are held in the intensity and directions that the light is scattered. However, lenses have a limited size, limiting the amount of light captured. The image that is reconstructed from the light captured by the lens will not have the details carried by the light that never reached the lens. Our image is imperfect.
For the finest of features, there is no angle at which a lens can capture the light, because the light doesn't travel. Instead, the wave dies rapidly (exponentially), and within a few wavelengths, the intensity is very close to zero. A lens, with a working distance typical of a microscope, will not capture these so-called evanescent waves.
A superlens is designed to capture these detail-holding evanescent waves. To enable that, the lens must be constructed from a metamaterial that has a negative refractive index (normal materials have a positive refractive index). However, metamaterials are not easy to make, and don't perform well. Most of the light that hits a superlens is reflected from it, while internally, the substances that are used to create the metamaterial absorb a lot of light. Hence, the lens captures fine details, but the image contrast is poor.
This is where the work of Song and coworkers comes into play. Their lens consists of an array of waveguides that are placed very close to each other. Each waveguide captures light from just in front of the waveguide opening. The light is transported to the other end of the waveguide array, where it is used to (in principle) recreate an image.
Waveguide flow control
Closely spaced waveguides don't transport images. When waveguides are close together, the light flows from one waveguide to another. An image will be completely randomized if it is transported in a dense array of waveguides.
To get around this problem, the researchers exploited how the coupling between the waveguides works. In straight parallel waveguides, the coupling between the arrays can be represented by a fixed positive number. This number gives the fraction of light that swaps waveguides as a function of distance. However, if the waveguides are parallel, but meander in a wave-like manner, then the coupling can be negative.
To be more concrete: imagine two waveguides that are close together and straight. Light enters one waveguide and spreads to the second at a rate given by the coupling constant. The light then enters the meander, which has a coupling coefficient that has equal size, but is negative. This section undoes the spreading exactly so all the light exits the same waveguide it entered.
The researchers demonstrated this effect with an array of 13 waveguides. They showed that light would consistently exit the waveguide it was coupled into, despite severe mixing in the straight section.
This is only the beginning of the story. Images can be built up by scanning the waveguide array. The resolution can be further increased by making the aperture of the waveguides smaller.
The demonstrated structure has other uses. Integrated optical circuits for computing and communications are, compared to electronic systems, large. The spacing is dictated by the need to control the coupling between neighboring waveguides. This research shows how to have high density waveguides without unwanted coupling. In the end, that could find applications more widespread than high resolution imaging.
(From:https://phys.org/news/2020-05-waveguide-array-distortion.html)
